Generally, isotopes have always been examined as parts of a family of same-element isotopes. This research was quite different in considering them instead to be parts of families of same-atomic-weight isotopes.
The possible error factors of the accuracy of the experimental numbers are such that we also know that there is also less than 1 electron-Volt of energy that could account for any neutrino that is also supposed to escape. But the accepted reasoning involves a central event in order for this decay process to occur, that a neutron inside the H-3 nucleus must break apart (decay) into a proton, that electron and that (anti-)neutrino (which was proposed in the 1930s entirely to try to explain how a neutron, having Spin of 1/2 could decay into a proton, having Spin 1/2 and an electron, also having Spin of 1/2. For some reason, those early Physicists assumed that Spin (Angular Momentum) was a SCALAR quantity and not the VECTOR quantity which it actually is! The fact that neutrons apparently do not actually exist within atomic nuclei seems to suggest that there could be no source for the multitude of neutrinos which most scientists ASSUME fill the Universe! Their reasoning clearly shows that there should have been 0.78235 MeV of neutron binding energy that would have to have been released PLUS whatever energy would have been necessary to CREATE the anti-neutrino PLUS whatever kinetic energy that anti-neutrino would carry away, per the conventional descriptions of nuclear processes. These are all logical contradictions to the Conservation of Mass / Energy and of the standard understanding of what occurs and these facts presented here are fully documented. Even if a neutrino is thought to have a zero rest-mass, SOME amount of energy must originally exist to provide it motion energy. Photons do not have rest-mass, but still there must be some initial energy that then exists as the measurable energy (radiation) of the photon. We just confirmed the amount of KINETIC energy that the electron carries away. With less than 11 electron-Volts available from Tritium decay, it is hard to see how a neutrino could be emitted from that specific Beta decay, or how it could have any energy related to motion. This tiny 1 electron-Volt can even be totally be accounted for. A NEW orbiting electron in the new Helium-3 atom would involve a tiny amount of orbital Kinetic Energy. There may also be a tiny amount of Kinetic Energy of the previous Migrating electron inside the previous Tritium nucleus, likely also just a few electron-Volts of energy. The math of the Conservation of Energy might easily be PERFECT.
There is also the matter of the Weak Nuclear Force, which is universally assumed to exist within atomic nuclei, regarding the occurrence of Beta decays and EC captures. THAT Force was ASSUMED to be required by those 1930s Researchers in order to try to claim Conservation of Energy / Mass. However, we have just seen that there is NO energy or mass which is not fully Accounted for, when neutrons do NOT exist within atomic nuclei. This seems to suggest that the Weak Nuclear Force might not exist within atomic nuclei, either.
Consider 7Be4, which decays with a half-life of around 54 days by the EC process. When the nucleus is considered to contain only electrons and protons and no neutrons, we can see that NO difference of mass occurs in this process. The result of this Electron Capture is 7Li3. It is experimentally determined that Radiation of 0.8618 MeV energy is released in this decay. If we examine the NIST atomic masses, we see that 7.0169292 AMU is converted into 7.0160040 AMU. This is a difference of 0.0009252 AMU mass which disappears. Converting this to MeV, this is 0.8618 MeV, which provides the energy of the radiation given off by the decay.
IF a newly formed neutron within the 7Li3 had required 0.78235 MeV to bind that electron to a proton to form the new neutron, then most of that available energy would have had to have been used up. But it certainly is NOT used up, and in fact, the energy accounting is impressively accurate in emitting radiation of exactly the amount of the mass difference of the initial and final nuclei.
This situation is also true for many other EC decays. 11C6 releases 1.982 MeV of radiation, which exactly accounts for the mass difference. Similarly for 40K19 (1.5048 MeV), 41Ca20 (0.4213 MeV), 49V23 (0.6018 MeV), 51Cr24 (0.7527 MeV), 53Mn25 (0.597 MeV), 55Fe26 (0.2314 MeV), 68Ge32 (0.106 MeV), etc.
Further proofs for neutrons not existing within nuclei are contained in the following text.
3H1 to 3He2 decay is discussed above, but research has found that essentially the same reasoning applies to virtually all other nuclear decay events. Those decays, whether Beta+, Beta- or EC, which alter the number of neutrons in a nucleus by one, never show any indication of accounting for the allegedly required neutron self-binding energy of 0.78235 MeV.
These two atoms have the same nominal atomic weight, but their precise weight is different. For 181Re75 it is currently known (NIST data) as 180.950065 AMU. For 181Os76 it is 180.95327 AMU. Since the actual components (considered as protons and electrons) are in the exact same numbers, this difference must therefore be completely due to differences in four things: (1) the binding energy involved in holding each of the atom nuclei together; (2) the binding energies holding the individual neutrons together inside the nucleus, actually the one neutron principle in this discussion; (3) the energy present associated with the neutrinos involved inside the individual neutrons inside the nucleus; and (4) the energy equivalent of whatever pions are present inside the nucleus.
According to standard Physics, then, there are a lot of different binding energies that must be included in this. The primary one, the first one listed above, is usually called the Strong Nuclear Force. It was first proposed in the 1930s, because it was recognized that there is incredibly powerful electrostatic repulsion between the positively charged protons in the nucleus. Left to themselves, those protons would repel each other out of the nucleus in a tiny fraction of a second. Because those protons are so physically close together, their repulsion is clearly (and easily calculated, as below) extremely strong, so they are clearly constantly trying to fly apart, which would cause every atom to decay into some other element. Since many isotopes are stable, the Strong Nuclear Force was postulated (in the 1930s) as the way atoms could be stable. Since the electrostatic repulsion is extremely strong and has an inverse-square dependence on distance, the Strong Nuclear Force was postulated as therefore being an inverse-cubed or higher distance dependence, to be extremely powerful at short distances between protons but to not have any measurable effect beyond the nucleus. In my Physics education at the University of Chicago, I was taught that it was an inverse-fifth-power dependence, although there seems no actual evidence to support such a specific claim, and such claims seem rarely made any more.
This Strong Force therefore represents a primary Binding Energy which was postulated in the 1930s to be holding nuclei together, overcoming the also strong repelling effects between any pairs of positively charged protons. (There was and is no actual experimental evidence for the existence of such a Strong Force.)
In addition to the Strong Nuclear Force binding energy, there must also be a binding energy that holds internal neutrons together. We already addressed this matter in the Tritium Beta decay mentioned above, where there is no possible energy related to the known internal binding energy of a neutron. Free neutrons have been studied regarding their natural decay and that binding energy is known to be around 0.78235 MeV (or 0.000841 AMU). There is also a very small factor in that there is one less electron orbiting the nucleus, so there is a difference in that ionization-related electron binding energy (rarely higher than 0.0001 MeV or 0.0000001 AMU). Conventional Physics also states that there are also many other binding energies described inside the nucleus, regarding various pions and neutrinos and other objects in the nucleus, as well as the energy equivalents of the rest-mass of such particles.
Therefore, that measured difference of NIST atomic weight MUST be due to a combination of these many contributions, but primarily due to the inverse-fifth-power (or similar) Strong Nuclear Force. This suggests that if we graph all the NIST atomic weights of isotopes of any one atomic weight, such as the 13 known isotopes of atomic weight 181, we should get a very complex graph. Below is that graph of the NIST data of the atomic weights of all the known isotopes of nominal atomic weight 181, straight from the NIST web-site, without any adjustments or corrections. Why is it that when we graph that NIST data there is such an obvious and amazingly pure parabolic shape? The first graph just shows the thirteen specific points representing the currently accepted (NIST) atomic weights of the isotopes of Element 70 through 82. The second graph is identical but also includes a best-fit parabola. The logic above, that of traditional Physics, suggests that this data should have extremely complex components, and probably contain a primary curve of an inverse-fifth-power shape. But it does not. In reality, it is remarkably close to being a simple parabola, with a statistical r2 of over 0.9992!
When a best-fit parabola is added
These graphs are representative of all same-atomic-weight isotope families. Some years back, I generated such graphs for every atomic weight where more than two isotopes are known. The group for 181 was chosen here because it contains many known isotope members. This provides enough data points to get a statistically reliable curve shape.
Consider for a moment that all 13 of the isotopes represented here have the exact same quantity of electrons and protons, if the constituent neutrons are seen as a combination of a proton and electron. Therefore, the amount of actual mass attributable to actual protons and electrons is identical in each case, and the different actual atomic weights are then purely due to differences in the binding energies, energy equivalents of pions, neutrinos, etc.
The three constants in the quadratic equation are useful numbers. a is the weight of the horizontal tangent line, that is the lowest possible atomic weight of that set of isotopes. It represents the absolute most stable nucleus for that atomic weight. Isotopes which are at the bottom of the parabola are always stable nuclei. c is the atomic number of where that most stable weight would be. It is generally between two actual integers of physically possible isotopes. b is a number that defines how WIDE the parabolic curve is, defining the semi-latus rectum.
This approach of examining same-weight isotopes has therefore eliminated the largest contribution to the weights, the actual mass of the components. Therefore, the effects that we see are necessarily due to whatever other objects or binding energies present in the nucleus.
It is noted that these graphs do not directly show any distance dependencies. But the purity of the parabolic shape seems significant. If there were strong binding energies due to a higher-distance-power Strong Force, it is believed that these graphs would reflect that. We will see later that the implications of these consistent second-power dependencies seems to lead to an astounding conclusion, that there must be an entirely new Law of nature in effect, one which is dependent on the square of the charge of a nucleus. But for now, we will continue with the analysis of the published NIST data.
This second-power dependence might suggest that a second-power Nuclear Force is the predominant cause that is providing the bulk of the nuclear binding energy. From the isotope at the bottom of the NIST data graph (which is always a stable isotope, as discussed below), as the number of surplus neutrons or lacking neutrons is doubled, the total binding energy of the atom changes by a factor of four. It seems reasonable to interpret this as an electrostatic effect, involving a second-power dependence.
In any case, the lack of any curve distortions due to any very strong binding energy source seems to deny the possibility of the Strong Force existing in nuclei.
In a simple graph of the NIST data which only appears to show second-order effects, where is any effect of the Strong Nuclear Force? It is hard to imagine that such an over-powering force as one which overwhelms the intense electrostatic repulsion, would not somehow show itself by dominant binding energies in such graphs. But there seems to be no indication whatever of any such binding energies. How can the Strong Nuclear Force actually exist?
However, when that attempted correction is made, for the universally accepted neutron-internal-binding energies, the data fit gets much worse! The statistical r2 value drops to 0.99885. That is still a tolerable curve-fit, but it is worse than the actual data! This seems to provide a third proof that the binding energy that holds neutrons together might not actually exist inside the nucleus. (Even more compelling mathematical evidence for this statement will be seen below.) Free neutrons certainly exist, and our experiments study them. However, this finding seems to suggest that they may not be actual neutrons inside the nucleus, that they may actually be distinct electrons and protons instead. Note that no significant energy seems to exist for the (allegedly) associated neutrinos either.
This shows up in every Beta or Beta+ decay, but it is most obvious in the Tritium (Hydrogen-3) decay into Helium-3, where the situation is quite simple and where the energy carried away by the escaping electron accounts for essentially all of the mass difference between the two isotopes (as discussed above). The fact that there does not seem to be any contribution in the total atomic weight for either the known neutron-internal-binding energy or any equivalent energy equivalent for neutrinos, seems to bring new questions into the picture.
In these many years of studying these subjects, I have now come to believe that there are actually no distinguishable neutrons INSIDE the nucleus, that the components exist as separate and independent protons and electrons. This view resolves the matter of there not being neutron-internal-binding energies or energy equivalents of neutrinos inside the nucleus. However, it has rather massive side implications regarding whether the Weak Nuclear Force even exists or whether neutrinos even exist.
Please note that I have NO grudge against the Strong Nuclear Force, the Weak Nuclear Force, or neutrinos! It is just that these simple graphs of pure NIST atomic weights are SO impressively parabolic that I have found it impossible to avoid these concepts.
It is hard to ignore what appears to be a 10-symmetry in the results. These findings may provide some data on internal structure of nuclei, with the apparent possibility of some sort of shells, similar to the way the electron cloud has its own 2-, 6- and 10-symmetries, since these various residual atomic weight graphs tend to have either a prominent 6-symmetry, a 10-symmetry or a 2-symmetry. There are also other patterns which seem to recur among the residuals charts. For example, if the atomic number and atomic weight are related by a simple fraction, there is usually a large preference (downward residual) (added stability for that isotope), while if either is a prime number, or if the two have no simple relationship, there is usually a strong negative preference (upward residual) (instability).
And would the alleged hundreds of nuclear neutrinos then be inside the nucleus or somehow outside of the nucleus that emitted a neutron?
In any case, given the compelling evidence that there are NO actual neutrons within atomic nuclei, then the case for claiming that neutrinos must also exist to conserve angular momentum when those nuclear neutrons decay, seems essentially beyond credibility. The implication seems to be that the entire reasoning for postulating neutrinos in the first place does not actually exist, and that there may not be any need for that postulization at all. There may not actually be any neutrinos.
If there is such a simple second-power (parabolic) term that describes the entire change in the total atomic weight (the entire change in nuclear binding energy), that seems to imply that only a second-power force must be acting. That seems to disagree with the higher-power Strong or Weak nuclear forces, and more directly seems to point to a possible electrostatic force acting.
Every family of same atomic weight isotopes has been examined, and the same prominent parabolic shape dominates every graph where there are enough family members for statistical validity. This seems to imply that a second-power effect within the atomic weight, directly related to binding energies, must be present, in all isotopes of all elements. Further discussion of this will be below.
From the same initial NIST data parabolic graph, there appears to be additional information that seems to be pretty reliable. The absolute value of the slope of the parabola (times a constant) at any given Element provides a surprisingly reliable prediction of the negative log of the half-life of that isotope. Near both ends of nearly every such graph, the slope becomes high enough to represent a half-life of around 10-23 second, probably about the shortest interval a distinct isotope could exist. (This is approximately the time it would take to cross an average nucleus at the speed of light.) This seems to then imply that the 181 family of isotopes is "nearly complete" toward the higher atomic number end but that there should be some currently undiscovered isotopes such as 181Tm69. There are several atomic weight graphs like this where the slope never gets that steep, which might suggest that certain specific isotopes might yet be discovered.
Near the bottom of each parabola, the longer half-life isotopes are always included, where the slope is lowest. For different atomic weights, there is sometimes an isotope near the very bottom of the parabola, which is ALWAYS stable. In this case, it is 181Ta73. The graph has very little slope there and there is no lower available isotope (of weight 181) to either Beta- or Beta+ decay into. The graph therefore predicts that 181Ta73 is stable, and it is. There are some graphs where two isotopes are about equally distant from the bottom, and these two are either both stable or both with relatively similar long half-lives.
The adjoining isotopes, 181Hf72 and 181W74 are at locations on the graph where the slope is low, which predicts they are unstable but that they have relatively long half-lives, with W being longer. This agrees with experimental evidence, where 181Hf72 has a half-life of 42.4 days and 181W74 has a longer half-life of 121.2 days. Where the slope is steepest, for 181Tl81 and 181Pb82, the predicted half-lives are less than one second, and that agrees with experimental evidence. This type of analysis suggests that the hypothetical isotope 181Tm69 should have a half life of maybe 2 minutes or so, and that around 4 MeV should be created when it Beta- decays. This analysis therefore predicts certain future discoveries of isotopes while also predicting that others will probably never be detected.
First, as expected, the graph of actual atomic weight (y) against nominal atomic weight (x), is essentially linear. When plotted against y = a + b * x, b is 1.0002129. This seems to suggest that an electron and a proton and nuclear binding energy must total 1.0002129 AMU or 931.64 MeV. Even more interesting are the residuals of such a linear graph:
When a best-fit parabola is added
This parabola is a surprisingly good fit, with an r2 of over 0.995. This seems to provide even more evidence that the binding energies inside nuclei are second-order phenomena.
Equally interesting are the residuals of this statistical analysis.
There appear to be distinct patterns in this data. At around atomic weight 60, 90, 135 and 210, there are prominent upward features. These atomic weights are separated by factors of 1.5 to 1. Could this be some indication of internal structure in the nucleus? There may be "preferred configurations" of numbers of nucleons that represent "complete shells" such as with the orbiting electrons. For now, this comment must remain merely a speculation, but the regularity of these features seems important, and seems to be related to internal nuclear structure.
In the graphs of different atomic weight families, the phase of the residual cycle seems to vary. There is probably a meaning in this, which has not yet been understood.
It seems worthwhile to attempt to analyze the second-order residuals graph above for any indications of evidence for "completed shells" and then examine the various residual patterns for different atomic weights to see if there is any mutual confirmation. Between the two, there might be good evidence of specific internal nuclear structures.
This graph is so irregular that it seems beyond mathematical analysis. In general, it seems to have been neglected in that regard, because of its complex shape.
It is the chart of the difference between the experimentally measured atomic mass (recently NIST data) and the sum of the known atomic masses of all the component parts. Traditionally, the number of protons in a nucleus was multiplied by the number of protons present; the number of neutrons was multiplied by the number of neutrons; and the number of orbiting electrons multiplied by their number, to get a grand total of mass which can be allocated to actual objects. The experimentally measured atomic mass is different from that (except for Hydrogen-1). That difference is called the Mass Defect and it is assumed to be the total of all binding energies and masses of any other particles inside the nucleus, such as pions and neutrinos. That quantity has traditionally been divided by the atomic weight, to get an amount of energy PER AMU. For nearly all stable isotopes, it is generally around 8 MeV, but there are complex variations as seen in the Mass Defect Chart above.
We can do example calculations for the traditional Mass Defect Chart. That sees the atom of the common form of Uranium as being composed of 92 protons (at 1.00727646 AMU each), 146 neutrons (at 1.008664916 AMU) and 92 orbiting electrons (at 0.000548 AMU). Adding these together, we get 239.984928 AMU, for the TOTAL mass of the component objects in AMU.
We then check the NIST data base for the experimentally measured mass of that atom, which is 238.0507812500 AMU. The DIFFERENCE is 1.934146806 AMU. We then multiply that by 931.44 to convert into MeV, and get 1801.5417 MeV. This is the TOTAL Mass Defect for that atom, and we then divide it by 238 to get 7.5695 MeV, the value shown in the traditional Mass Defect Chart above. You can see that this value is shown at the end of their graph line.
This research has discovered some interesting new insights regarding
the Mass Defect! For one thing, if it is considered as THREE overlapping
charts, one for ODD weight atoms, one for even-even weight atoms and
the third for odd-even atoms, the three charts are each then much simpler.
Our three separated graphs show a slightly lower value, because we did not include neutrons as neutrons but as separate protons and electrons. Therefore the (alleged) neutron binding energies are not actually in there, and so we left them out, causing slightly lower graph lines.
However, the Mass Defect Chart seems to provide impressive evidence that the premise of this research, that free-ranging electrons exist within atomic nuclei, is valid. If we present these same three component Charts, but instead of dividing the total Mass Defect by the total weight of the atom, we divide it by the NUMBER OF MIGRATING ELECTRONS IN THE NUCLEUS, we get surprising new information!
Notice that we now see short series of atoms in relatively linear patterns. These are actually groups of atoms which have the SAME NUMBER OF EXCESS MIGRATING ELECTRONS. For example, in the first of our three Charts, the one which includes ODD weight atoms, there is a distinctive pattern of the atoms which have 49, 51, 53, 55, 57, 59, 61, and 63 total nucleons. These atoms all have FIVE Excess Migrating Electrons in their nuclei, or what would have traditionally been said to have been "five more neutrons than protons". Just to the right of that, we see a grouping of three which have SEVEN Excess Migrating Electrons. And then a group of five that have NINE; and then an interrupted group of nine which have ELEVEN, and so on. You might even notice that the groups are even spaced apart in a consistent manner. These are all clues to details of nuclear structure!
The amounts of energy shown here are somewhat greater. The TOTAL Mass Defect is simply divided by a smaller number of objects! For the common form of Uranium, we know that there are 92 electrons orbiting the nucleus and a total of 238 nucleons inside the nucleus. This research is suggesting that we should look at that nucleus as being composed of 238 protons and (238 - 92) 146 migrating electrons. The entire atom then contains 238 protons plus two sets of electrons, 146 of them migrating INSIDE the nucleus and 92 of them orbiting AROUND the nucleus, for a total of 238 electrons. This balance keeps the atom electrically neutral.
We know the mass of a single proton and also of a single electron. They total 1.007825032 AMU. So if we multiply this number by 238, we would have the total mass that is attributable to the actual objects involved. This amount is 239.862357616 AMU.
We can then check the NIST data base for the experimentally measured mass of that atom, which is 238.0507812500. The DIFFERENCE is 1.811576366 AMU. We then multiply that by 931.44 to convert into MeV, and get 1687.37469035 MeV. For our modified Mass Defect Charts, this quantity would be divided by 146, to get 11.5573 MeV per Migrating electron.
But it suggests that the long-assumed complexity of nuclear structure may not be any more complex, or even different, from the long-studied behaviors of orbiting electrons. THIS seems enormous! It might imply that two very different fields of nuclear Physics may actually be the same subject!
A rather simple second power equation:
For the ODD atomic weights graph, the values currently calculated for the constants are: k1 = 0.12158; k2 = .0080342; k3 = .0000096544; and k4 = 126.46. k3 and k4 are directly from the 'best weights' residuals parabola above (b and c in that equation). k1 is a composite of constants from the linear and parabola equations. k2 is related to the relationship between an AMU and a the mass of a proton plus electron.
Slightly different constants apply for the other two graphs.
The equation above shows a maximum binding energy per nucleon at around atomic weight 56 or 58. This agrees with the standard Mass Defect Chart shape. This may be a confirmation of the general impression that the nucleo-genesis within supernova stops once Nickel-56 is formed. It may represent a theoretical reason why nucleo-genesis of higher atomic weight nuclei does not occur.
There is an extra step that is necessary for even-atomic-weight
family isotopes, and it appears to provides information regarding
another insight into nuclear structure. In this case, two separate
graphs need to be made, one for the even-atomic-number isotopes
and the other for the odd-atomic-number isotopes.
The actual reason these are different is that they have different numbers of Excess Migrating Electrons from each other.
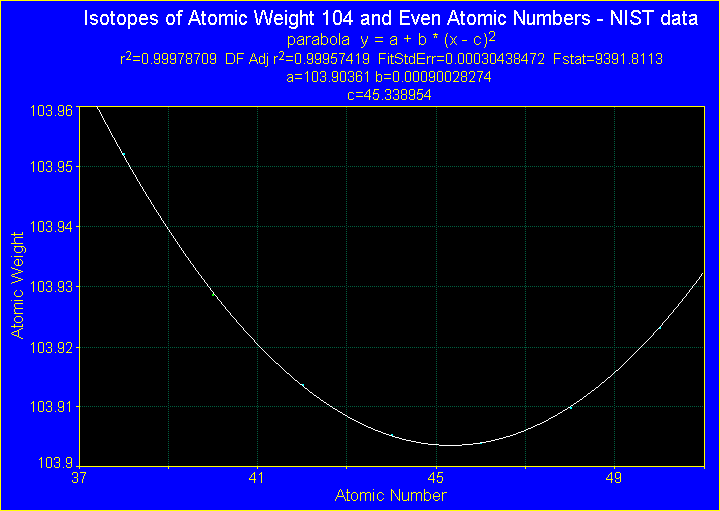
Each of these graphs is a very good parabolic shape, having
r2 values of 0.999787 and 0.999109, respectively, each much
better than the 0.99121 of the combined graph above. An important value
in each of these graphs is the least value of the parabola weight,
103.90361 and 103.90631, respectively. These values are different
by 0.00270 AMU. An interpretation is that an even-even symmetry
(atomic weight / atomic number) has a structural advantage of
stronger bonding (or better nuclear stability) than an even-odd
symmetry, by an amount of 0.00270 AMU. With this assumption, we
might adjust the weights of the even-odd isotopes downward by
0.00270 to account for such an advantage of the even-even isotopes.
In this case, if we now graph the entire isotope family again,
we get a parabolic shape comparable to those of odd-atomic-weight
isotope families.
This graph has an r2 of 0.999157, indicating a very good parabolic fit. This seems to confirm the advantage of 0.00270 AMU for nuclei which have an even-even configuration over that of an even-odd configuration. This seems to explain why we find that stable isotopes are extremely rarely even-odd isotopes (Nitrogen-14 is nearly the only common example) and are very commonly even-even isotopes. The discussion below will suggest that an even-odd isotope might actually contain two odd quantities (of protons and of electrons) which might account for an extreme difficulty regarding mechanical symmetry and therefore stability.
Each of the hundred-plus even-atomic-weight family graphs seems to have a differential which is close to 0.0027 AMU. The fact that the same differential appears in all of those graphs and in none of the odd-atomic-weight family graphs seems extremely compelling and important.
There are several additional smaller factors which modify the precision which those parabolas give. The most prominent and consistent appear to be two 2-symmetries, which result in a natural stability preference for even-atomic-weight and even-atomic-number isotopes. The two-symmetry for atomic number appears to be on the scale of 1/3 MeV, while the two-symmetry for atomic weight appears to be around ten times that large, around 2.5 MeV. There appear to also be smaller 6-symmetries and 10-symmetries, for each, which slightly affect the final precise atomic weights. These factors appear to be very consistent, and simple terms may be added to the equations above to make they even more precise.
This new analysis appears to offer theoretical bases for many phenomena regarding nuclear structure, isotopic stability, and nuclear reactions. For example, it seems to theoretically explain/predict that odd-atomic-weights generally have only one stable isotope while even-atomic-weights generally have two (with a meta-stable isotope in between). Even the rare exceptions are seemingly explained. A theoretical basis seems to be provided for the approximate half-life of all isotopes, including the ones that are stable. There is a theoretical implication that virtually all possible isotopes have already been found and measured, with a few specific unfound isotopes to possibly search for.
The conclusion seems unavoidable that such simple second power terms are capable of very accurately defining the actual precise atomic weight of any isotope. That seems to imply that only second-power effects are acting, with the primary suspect being the inverse-square electrostatic force. This presentation is meant to demonstrate some examples of the analysis that resulted in the second-power equations, and then suggest a physical electrostatic mechanism that might be acting. If this premise is valid, then nuclei might be shown to be stable or unstable exclusively on the basis of electrostatic forces. No Strong Nuclear Force would need to exist to explain nuclear stability.
The exact same reasoning is applied to planets orbiting the Sun, where their kinetic energy of revolution exactly matches the amount of potential energy that would need to be added to the planet to move it out of the Solar System to an infinite distance. These are consequences of the Conservation of Energy, whether in electrons orbiting a nucleus, planets orbiting the Sun, or satellites orbiting a planet. The calculation involved is often referred to as the Hamiltonian.
So now consider an electron, but one that falls into a far closer location than orbiting electrons have. We will just consider ball-park numbers here to show a general concept. We know that the electron orbiting a Hydrogen nucleus has around 13.6 eV (electron-Volts) of kinetic energy, and that it has a -13.6 eV amount of potential energy (when referred to infinity) We know that results in a radius close to the First Bohr Radius, of 5.29 * 10-9 cm. This is a diameter of about 1 * 10-8 cm.
We know that electrostatic situations have an inverse square force law, which causes an inverse first power law regarding potential energy. So if we would halve the radius of the orbit, the potential energy converted to kinetic energy would be two times as great. This presentation suggests that maybe we should consider the Migrating electrons INSIDE the nucleus identically to how those orbiting electrons are considered.
We will see below that there appears to be around 1.3 MeV of energy which may be associated with each Migrating electron inside the nucleus. That is around 100,000 times as much potential energy converted to kinetic energy as for the orbiting electron in a hydrogen atom. That seems to suggest that we might consider the electrons INSIDE the nucleus, in an entirely electrostatic way, using conventional electrostatic formulas (except for the fact that the velocities are relativistic, so those adjustments need to be applied). The comments above then suggest that we would be talking about an "orbital radius" of 1/100,000 the size of the orbiting electron's orbital radius. That would be an orbit diameter of around 10-13 cm. This is in good agreement with the believed diameter of the nucleus of some atoms, and in the dimensions of a neutron. If this is so, then the distinction between electrons orbiting the nucleus or orbiting INSIDE the nucleus may be somewhat insignificant.
My studies during the past several years have suggested the possibility that simple electrostatic attraction and repulsion of protons and electrons inside the nucleus might describe everything that is detected in experiments. This will be discussed below.
After studying this data, these graphs and their implications for more than four years, I now find it hard to deny an electrostatic force as virtually the exclusive cause of binding energies within the nucleus. I have rigidly attempted to avoid making any assumptions that might damage this analysis.
The prominent parabolic shape of such graphs suggests a seemingly logical explanation of nuclear stability without having to require a Strong Nuclear Force. This new perspective suggests that simple Coulomb electrostatic forces may offer a straightforward explanation of nuclear stability, and even possibly explain many aspects of variations such as predictions and calculations regarding radioactive decay.
A suggested situation is similar to the structure of a crystal where positive and negative ions are maintained in a stable arrangement due to a "lattice energy", which is entirely an electrostatic phenomenon. Reasoning very similar to the electrostatic arguments regarding orbital electrons and regarding interactions between atoms, particularly in crystalline structures, might be applicable. It also includes the possibility that the Heisenberg Uncertainty Principle might permit the electrons within a nucleus to seem to be at various specific locations as appropriate. The following argument seems to provide compelling evidence that the Strong Nuclear Force is not necessary in keeping nuclei in stable arrangements. The evidence below seems to suggest the possibility that atomic nuclei may actually be composed of 'A' protons and 'A - Z' somewhat free-ranging electrons.
This pattern provides equal distances between the protons/nucleons. Even if
the entire tetrahedron would rotate, the tetrahedron structure seems
likely to persist.
Such a large repulsive force would clearly cause the two protons to accelerate away from each other, outward, disrupting the integrity of the nucleus. Therefore, it had been concluded as early as 1935 (by Hideki) that no conventional Physics explanation could explain why a nucleus would not immediately fly apart. The solution given was that there MUST BE a Strong Nuclear Force, which is attractive and which has a distance dependence that is far higher than the inverse square dependence of the electrostatic (Coulomb) repulsion. Its attraction at the short ranges within the nucleus would therefore overcome the electrostatic repulsion of the protons to keep the nucleus together.
This Strong Nuclear Force would have such a short effective range of action that it would never have any significant effect outside the nucleus, and therefore not alter any other physical actions. The Strong Nuclear Force was therefore INVENTED to try to explain a situation that did not seem explainable in any other way. But the Strong Nuclear Force has never had any theoretical basis of existence, except for the fact that it "must" exist to counteract the mutual electrostatic repulsion of the protons. There has really never been any compelling experimental evidence that it actually exists. However, virtually all of modern particle physics is greatly based on it. It seems to be accepted because no one has ever seen cause to question its validity or reality.
The analysis of the NIST data above seems to argue against the existence of a Strong Nuclear Force. The data does not seem to show any higher- order attraction contribution to the total mass of atoms, and there is not even any contribution to the total mass from the pions which are believed to carry the Strong Nuclear Force inside nuclei. This seems to present a problem.
It might be noted that no significant assumptions were made in any of the above discussion. The universally accepted NIST data was simply graphed in a specific way, standard statistical analysis was applied, and the results were considered. This seems to be in marked contrast to the hundreds of speculative assumptions that seem to regularly be applied today within Physics. I am sometimes ashamed at the outrageousness of some assumptions that are put forth, and then generally accepted. What has Physics come to?
This would initially seem to make the situation even worse! Now we
are considering FOUR positively charged protons each repelling
each other with extremely powerful forces! On first glance, this
might seem even less stable. But that may not actually be the case.
Consider the situation where one of those two electrons is momentarily at a point halfway along the line joining two of the protons, exactly at a midpoint of one of the edges of the tetrahedron in our drawing. For the moment, ignore the other components of the nucleus and just consider these three objects, proton, electron and proton, which are equally spaced along a straight line.
One of the protons and the electron each have opposite electrostatic
charges of 4.80294 * 10-10 electrostatic units, and they
are approximately 5 * 10-14 cm apart (half the previous
distance). Therefore, they electrostatically attract each other with
a force of
This electrostatic attractive force is four times stronger than the repelling force (calculated above) that still exists between the two protons. This would result in a NET ATTRACTIVE FORCE acting on each proton of three times the original electrostatic repulsion of the two protons (+4 -1). This is equally true for each of the two protons involved. Therefore, the resulting effect would not be of the protons flying apart, but actually being more likely to want to accelerate toward each other, actually toward the electron, with extremely high acceleration! This simple straight line arrangement would quickly collapse toward each other!
It might be pointed out that the attractive forces acting on the electron in this specific situation are exactly equal and opposite, and as long as it remained exactly at the centerpoint of the line between the two protons, it would therefore experience no acceleration, even though its inertial mass is much less than either proton. The electron would be in a meta-stable situation.
We must now consider that this particular nucleus has six such
center-point locations for the electrons to occupy to enable this
effect, but there are only two electrons. The premise here is that
if an electron constantly stayed at any such center-point, the attractive
force would be too strong, and it would quickly pull both protons
toward it and nuclear instability would result. However, if the
two electrons rapidly migrate back and forth among those six
locations, they could reside in each center-point location
for nearly 1/3 of the time.
This (animation) drawing suggests how the two electrons might occupy the six locations for equal intervals of time, essentially 1/3 of the time at each location.
This situation would result in each pair of protons electrostatically
repelling each other for 2/3 of the time, but then the presence of
the electron at that center-point would cause an attraction that is
three times as strong for the remaining 1/3 of the time, resulting
in a net effect of an overall electrostatic attraction. Since
these cycles would occur extremely rapidly, the averaged effect
would be a time Integration of these effects. The result (in a stable
nucleus such as He-4) would be an exact matching of attractions
and repulsions and therefore of a nuclear stability and clearly the
protons would not be exiting the nucleus.
These assumed oscillations might have some external effects. At a point where a nucleus became unstable, where an electron or proton left, the frequency might determine the frequency of the radiation emitted.
It is also certainly true that instantaneous electrostatic attractions and repulsions from the other components of the nucleus make the calculations more complex. Also, the protons themselves may be moving around, and the distances between them would certainly be variable as the various oscillations were occurring. The electrons might require some migration time to get from one centerpoint to the next. A statistical analysis of time-averages is necessary to determine the net effects on each constituent part of the nucleus. For heavier atomic nuclei, this quickly becomes very complex mathematically. The premise suggested here is that such or similar effects would eliminate any apparent net attractive or repulsive electrostatic force on each proton and provide at least a meta-stable neutral force on each, providing nuclear stability.
Known parameters of atomic nuclei provide a guideline regarding how rapid such migrations might occur. We know that atomic nuclei are on the order of 10-13 cm in diameter. A proton has a mass of 1.65 * 10-24 gm. We calculated above the force of electrostatic repulsion, at 2.3 * 10+7 dynes. Assuming non-relativistic motions, and for minimal variations in the distances involved, F = m * a or a = F / m, will give an approximation of the acceleration of the proton. This solves to an acceleration of 1.4 * 10+31 cm/sec2.
We might consider an absolute limit of an individual proton's movement due to Coulomb repulsion from another proton, to be half the nucleus diameter, or 5 * 10-14 cm, if nuclear stability is to be maintained. Again assuming non-relativistic velocities, then d = 1/2 * a * t2 or t2 = 2 * d / a or t2 = 7 * 10-45 or t = 8.5 * 10-23 seconds. This value is not particularly precise because we did not consider the variable force due to the variable distances that exist, and did not consider the relativistic effects of the extreme velocities of the movements of the migrating electrons, but it is only meant to give a ballpark idea of the time involved for each of the two repulsion portions of this cycle. The entire cycle would then be three of these time intervals, on the order of 3 times that long or 2 * 10-22 seconds.
This reasoning uses the longest likely interval that the proton-proton repulsion could be in effect without the electrostatic repulsion destroying the integrity of the nucleus. Therefore, it represents a guide to the longest possible cycle time for the process described above. As long as the electrons complete their entire migration path cycle in a shorter time than this, then the protons would not be de-stabilized, although they would likely experience a cyclic oscillation at that rate. If the cycle occurred more rapidly than that, the movements of the protons would be smaller and stability would be greater.
This situation suggests that the electrons, if described as moving, would need to traverse a cycle of three segments, or around 2.5 * 10-13 cm in a period no longer than 2 * 10-22 seconds, which implies a minimum velocity of around 2 * 10+9 cm/sec, about 1/15 the speed of light. This is interesting in that, should it be a higher velocity, then relativistic velocities of the electrons would increase their mass and possibly affect the reasoning regarding the mass defect and many other effects.
Consider this situation where one of those two electrons is momentarily at the exact centerpoint of a tetrahedron face which joins three of the protons. Again, for the moment, ignore the other proton on the other side of the nucleus and just consider these four objects, three equally spaced protons in an equilateral triangle formation, and the electron, exactly centered in that triangle.
Any one of the protons and the electron each have opposite electrostatic charges of 4.80294 * 10-10 electrostatic units, and they are approximately 7 * 10-14 cm apart. Therefore, they electrostatically attract each other with a force of
This electrostatic attractive force is about two times stronger than the repelling force that still exists between each pair of protons. This would result in a NET ATTRACTIVE FORCE acting on each proton of roughly double the original electrostatic repulsion of the pair of protons. This is equally true for each of the three protons involved. Therefore, the resulting effect would not be of the protons flying apart, but actually being more likely to want to accelerate toward each other, actually toward the electron, with extremely high acceleration! This simple arrangement would tend to quickly collapse that triangular face of the tetrahedron!
It might be pointed out that the attractive forces acting on the electron in this specific situation are exactly balanced, and as long as it remained exactly at the centerpoint of the face between the three protons, it would therefore experience no acceleration, even though its inertial mass is much less than any of the protons. The electron would be in a meta-stable situation.
It might also be possible that some electrons migrate between edge centerpoints and other electrons migrate between face centerpoints. In certain isotope configurations, one or the other might represent a more stable process.
Extending this premise one step further, consider the tetrahedron pattern of four protons to all be at a constant radius from the geometric center of the nucleus, with two migrating electrons generally occupying locations at slightly less radius. That would represent a sub-shell of nucleons that we might refer to as an (s) subshell. There are four protons involved, but because of the two migrating electrons also in the sub-shell, the effect might be perceived as a 2-symmetry.
The tetrahedron is one of five regular polyhedrons. These structures are each shell-structures where everything in the shell is at a specific radius from the center of the nucleus. Specifically, an icosahedron has 12 vertices and 20 surfaces, and a dodecahedron has 20 vertices and 12 surfaces. Both have 30 edges. An icosahedron structure could represent a sub-shell which we might call a (p) subshell. It would have a maximum of 12 protons located at its vertices (akin to our earlier analysis of the tetrahedron shape). Migrating electrons might intermittently visit either the 30 edge centerpoints or the 20 surface centerpoints, per the two possibilities discussed for the tetrahedron. In either case, this could specify the number of migrating electrons necessary for a complete (p) sub shell. If the edge-centerpoint premise is assumed, there may then be 6 migrating electrons visiting the 30 edge centerpoints between the 12 fixed protons, for the maximum stability of a complete (p) subshell. This might previously have been described as six protons and six neutrons, and the net effect might then be perceived as a 6-symmetry.
A dodecahedron structure could represent a sub-shell which we might call a (d) subshell. It would have a maximum of 20 protons located at its vertices (again, akin to our earlier analysis of the tetrahedron shape). Migrating electrons might intermittently visit either the 30 edge centerpoints or the 12 surface centerpoints, again per the two possibilities discussed for the tetrahedron. In either case, this could again specify the number of migrating electrons necessary for a complete (d) sub shell. If the edge-centerpoint premise is assumed, there may then be 10 migrating electrons visiting the 30 edge centerpoints between the 20 fixed protons, for the maximum stability of a complete (d) subshell. This might previously have been described as ten protons and ten neutrons, and the net effect might then be perceived as a 10-symmetry.
This might then provide an actual physical and mathematical explanation for the 2-symmetries, 6-symmetries and 10-symmetries that are found within the nucleus of various isotopes, as was observed in the Residual Graphs shown above. Various atomic weights analysis of NIST data shows various prominent 2-symmetries, 6-symmetries and 10-symmetries. This reasoning might provide a theoretical basis for their existence in the nucleus.
The mathematics seems to suggest specific radii for each of these various sub-shells, all sharing the same nucleus centerpoint, but as shells of various radii around that point. For a heavy nucleus, this would suggest that nearest in, there would be a small (complete) tetrahedron of four protons and two migrating electrons, as a 1s subshell. Surrounding that would be a larger (and therefore lower binding energy) tetrahedron as the 2s subshell. Surrounding that, an even larger icosahedron as the 2p subshell. Surrounding that, an even larger tetrahedron as the 3s subshell. Then a larger icosahedron as the 3p subshell. Next a larger dodecahedron as the 3d subshell. Surrounding that, an even larger tetrahedron as the 4s subshell. Then a larger icosahedron as the 4p subshell. Next a larger dodecahedron as the 4d subshell.
This already describes more than half the elements. It is important to consider the atomic weight as critically important over the atomic number in this description. In other words, an isotope of atomic weight 4 (such as natural Helium) would be a complete 1s subshell. At isotope of atomic weight 8 (such as Beryllium) would then represent a filled 2s subshell, and an atomic weight of 20 (such as natural Neon) would represent a filled 2p subshell or a filled 2 shell.
Continuing, atomic weight of 24 (such as magnesium) would represent a filled 3s subshell, and atomic weight of 36 (such as a natural Argon isotope) would represent a filled 3p subshell and possibly a completed 3 shell.
This reasoning is still currently incomplete regarding exact details of the icosahedron and dodecahedron structures, regarding what the ideal number of migrating electrons might be, as it depends intimately on whether the electrons migrate to the edge centerpoints or the face centerpoints. Therefore, the 1:2 proportion of electrons to protons would not necessarily hold and more electrons may be necessary due to the more complex geometry. Experimentally, this is seen, where heavier nuclei are all traditionally described as having additional neutrons in them. In this description, that statement would be described as involving a larger number of migrating electrons to adequately service the many centerpoint locations.
These ever-larger enclosing tetrahedrons, icosahedrons and dodecahedrons seem to provide a description of the majority of all nuclei and isotopes. However, this MIGHT require a modification of a long-standard method of description. We generally refer to Hydrogen and Helium to represent the first line of Mendeleyev's Chart. That may not technically always be true. An isotope like Hydrogen-6 or Helium-6 might actually require that two of its protons be in a 2s subshell instead of the 1s. If we only discuss stable nuclei, then the Mendeleyev Chart seems sufficient. If we also want to include isotopes, then sometimes an isotope may more correctly belong to a line above or below its normally accepted location in the Chart.
There does seem to be a problem that is not yet understood. There may be 14-symmetries in the nucleus as are seen in electron subshells. I have never seen compelling evidence of any 14-symmetry in any of the NIST analysis charts. But if a 14-symmetry exists, then that seems to imply a geometric regular polyhedron which does not exist. As a pure guess, I am tempted to wonder if a 14-symmetry might actually be a combination of a dodecahedron 10-symmetry and a cube 4-symmetry. But I have not seen any evidence to support such a statement.
THIS seems to be a potential significant advance. It is an indication (and also mathematical calculations) which identify a best number of neutrons for each atomic weight. In contrast, conventional reasoning that neutrons are present in the nucleus as NEUTRAL particles, there is an implied value in adding greater numbers of neutrons to provide the best stability. I have never seen any good explanation of WHY that is not experimentally seen. THIS approach not only gives good theoretical explanation, but even mathematical basis for determining just what that ideal number of (neutrons) migrating electrons needs to be.
This is the premise: We have always considered any atom as being made up of some number of protons and another number of neutrons inside a nucleus, with free electrons revolving around them (like planets) in a number exactly the same as the number of protons. If we now consider those neutrons to actually each be separate protons and electrons, then we now have a total of (protons plus neutrons) protons, all inside the nucleus; and a total of (electrons plus neutrons) electrons, some inside the nucleus and some orbiting it. The two totals are always exactly the same, matching the Atomic Weight number. But only the neutrons number of electrons are INSIDE the nucleus.
This then implies that as to actual massive objects, we have W protons plus W electrons. We have long known that the total actual mass of a proton and electron is the Atomic Weight of the Hydrogen-1 atom, or 1.007825032 AMU. Note that we are NOT including ANY Binding Force at all. However, the migrating electrons must have enough kinetic energy to move at relativistic velocities to get back and forth between their required locations. I currently believe that, per Hamiltonian concepts, the kinetic energy of those electrons came from potential energy as an electron fell from an infinite distance to its location inside the nucleus, very much following the accepted reasoning regarding orbiting electrons energy audit.
In careful examination of the NIST data, I have discovered that approximately 0.0133976 AMU of energy appears to be involved, although that exact number seems to depend on the diameter of the specific sub-shell involved and whether that sub-shell is filled or not. This may be due to the migrating electrons having to travel slightly different distances in fulfilling the total distance that each migrating electron must travel during one cycle for that specific nucleus.
In any case, take the atomic weight of an atom and simply multiply it by 1.007825032 AMU, to get a basic total atomic weight for that nucleus. There is then a small correction factor, apparently quadratic, which then must be applied to adjust this total weight. Subtract this number from the current NIST value for the atomic weight. This premise suggests that the resulting number should be the TOTAL kinetic energy of ALL the migrating electrons in that nucleus. If that number is divided by the number of those migrating electrons, we get a number which should describe the AVERAGE kinetic energy for the electrons in that nucleus. This Simple Calculation results in the following graph (for Odd Weight atoms):
This seems amazingly consistent regarding the suggested amount of kinetic energy per migrating electron among all the atomic weights! That energy is around 12.5 MeV per migrating electron, which suggests that the migrating electrons must travel at very close to the speed of light inside the nucleus. The data points for this graph and the one just below.
We can see additional factors regarding Fine Structure if we expand the vertical scale of this graph:
We can see that extremely consistent patterns appear to exist in this data. There are a number of groups of atomic weights where the amount of energy consistently becomes lower, meaning more stable. My current opinion regarding these patterns is that they represent some sort of sub-shells which become filled. At the point that such a sub-shell is filled, an additional migrating electron apparently becomes necessary. Each angled line segment represents nuclei with a consistent number of "excess neutrons" or possibly more accurately, "excess migrating electrons".
The sequence from Atomic Weight 49 to 63 seems especially interesting and will be examined more thoroughly below. These nuclei all have five more migrating electrons than would be double the Atomic Number, what I call Excess Migrating Electrons.
It turns out that examining the Odd Weight nuclei has a simpler graph pattern than examining the Even Weight nuclei. In the Even graph, there appear to be two separate patterns which seems to be due to some additional electrostatic effect. The Even-Even weights (8, 12, 16, 20, 24, etc) have a graph that is very similar to the Odd graph above. The Semi-Even weights (6, 10, 14, 18, 22, etc) also have a very similar graph. There is a vertical offset between the two, apparently implying that Even-Even nuclei may be more stable (most negative value here) where the migrating electrons may be slightly deeper inside the nucleus. Extensive research on this matter is needed.
These graphs have line segments added which connect isotopes which have the same number of excess migrating electrons. The groups to the right in the graph have additional excess migrating electrons, up to about 57 at the very rightmost end of the graphs
These graphs give a hint regarding the treasure of nuclear fine structure information which is available here, but the following three graphs seem must more impressive in that regard. This is focusing on the range of 48 to 63 atomic weight, where there seem to be extremely strong patterns shown.
The data points for this graph and the two
just below. In looking at the data for these weights,
it becomes clear that the reason for the irregular shape of the
patterns is due to the fact that the Odd weights have FIVE excess
migrating electrons, the Even-Even weights have FOUR, and the
Semi-Even weights have SIX. We can see that each of those three
patterns has a very consistent pattern. The following graph is the
same, with three line segments which identify the Semi-Even, Odd,
and Even isotopes in this range from Weight 48 to 63.
The following graph is again the same, but with the 16 isotopes, from Weight 48 to 63, grouped in sets of four, which alternate among the FOUR, FIVE and SIX excess migrating electrons families, with virtually identical resulting patterns.
There is yet another possible pattern of importance here. Again looking at the raw data, we can see that weights 50, 51, and 52 all have 28 migrating electrons; weights 54, 55, and 56 all have 30 migrating electrons; weights 58, 59, and 60 all have 32 migrating electrons; and weights 62 and 63 have 34 migrating electrons. (weight 64 has 36.) These are essentially the short line segments marked in the set of three graphs above.
We can note that each set of those groupings again has a very prominent nearly-straight line pattern with a very consistent slope (which was seen in the set of three graphs above which had those line segments added.)
A full understanding of the implications of these many patterns may take years of further examination. There seem clearly to be structural implications, where even or odd numbers of protons or migrating electrons have some stability advantages or disadvantages.
This is an impressively consistent value for the energy content. But if we greatly expand the vertical scale of the graph(s), we can see some fine detail as well.
First is the raw data directly from the NIST database:
A simple quadratic equation is then added with yet another parabolic curve!
This curve is rather accurate in having an r2 = .999543 The residuals from this curve's accuracy are then:
But the analysis presented here seems to include many nice insights into this subject. IF the tetrahedron concept has any merit, then there are actually TWO different explanations for why Helium-4 has zero spin! The traditional thinking is that the four nucleons are somehow always aligned where two are exactly opposite the other two, all somehow aligned in space with some reason for being mutually parallel. Fine. But our tetrahedron seems to offer an explanation which seems far more logical. What if all the four spins are aligned TOWARD THE CENTERPOINT OF THE TETRAHEDRON? When analyzed as VECTORS, the four spins then add to zero in all directions! In fact, this general concept seems to apply to ALL nucleons which contain an even number of nucleons, that is, an even atomic weight. There are very few even-atomic-weight isotopes which have non-zero nuclear spins, and they are all integer numbers. All the isotopes which have non-zero and non-integer spins (there are only around 60 of them now known) have odd nuclear weights. We see this as being a result of INCOMPLETE tetrahedrons and/or asymmetric icosahedrons that are also incomplete. Only a limited effort has yet been made in researching this area, but it seems that the nuclear spin can generally be predicted from the atomic weight and the number of Migrating Electrons in the nucleus.
P
/ \
P - E E - P
\ /
P
The geometrical arrangement would need to be such that the attractive and repulsive forces on each of the six objects are equal and at least meta-stable. This concept seems to become more problematical for large nuclei, as the fixed location electrons would seem to be less likely to be able to apply all the necessary attractions to stabilize all of the nucleons.
The fact that certain total numbers of nucleons might geometrically reside in particularly symmetric arrangements might explain the greater stability of some elements and isotopes than others (Including explaining some of the preferred residuals discussed above). For example, it could be that the number of nucleons that would otherwise be stable for Technetium might be an inherently unstable configuration.
This line of reasoning also provides a possible explanation for the fact that the vast majority of stable atoms are described as having even numbers of neutrons. This reasoning would suggest that an odd number of neutrons would imply an odd number of internal (free-ranging) electrons, which would possibly require much more specific geometrical requirements for the number and arrangement of the nucleons to provide a symmetry that is conducive to nuclear stability. An isotope that has an even atomic weight and an odd atomic number would therefore have an odd number of both protons and electrons in the nucleus. This seems like a credible reason why extremely few such isotopes are stable, Nitrogen-14 being the notable exception. If either the number of internal electrons or the number of protons is an even number, geometric symmetries seem more likely, and therefore better nuclear stability. If both the number of internal electrons and the number of protons is an even number, there seems to be even more potential for geometric symmetries, and this seems to give a theoretical explanation for why the vast majority of stable atoms happen to have such an even-even characteristic. The combination of the stability advantages of the two independent symmetry sources provides extremely stable nuclei. Other considerations along these lines will be discussed regarding the graphs below.
Most symmetry arguments can also be applied to the migrating nuclear electron premise described above. An even number of neutrons would mean an even number of migrating electrons, which might then act in concert in symmetric manners to establish especially stable nuclei. Along this reasoning, if both the number of protons and the atomic weight is even, the nucleus would be especially stable for having a double symmetry, so much so that an isotope of equal atomic weight and atomic number either one higher or lower is generally unstable with Beta decay. This situation is generally seen to be experimentally true.
As the electron would proceed from being joined with (or near) one proton or centerpoint to the next, it would travel some path between the two locations, not necessarily a straight line. The cumulative (Integral) electrostatic Coulomb attraction force between each proton and the electron can be calculated, if assumptions regarding the electron's path and velocity profile are included.
There are a variety of ways the electrons might actually move within the nucleus. A more generalized form of the above argument involves taking the time integral of the attraction between each of the protons and the electron during whatever path is followed. For most geometries of electron movement, the resulting effect is a slight reduction of the net attractive force. The initial (migrate) argument above would have had too much attractive force for stability, and effects such as this might ensure that the time-average of the attraction exactly equals the time-average of the proton-proton repulsion, in making a stable nucleus. A discussion below will consider the situations where there are more or less than an optimal number of electrons within the nucleus, and the effects on stability, on the half-life and the radioactive decay schemes.
However, this situation needed to be mentioned because it could occur for portions of the time cycles in the first premise suggested above. For example, for the Helium example discussed, consider if each electron resided bonded to each proton for 1/6 of the cycle, followed by an equal period of being at a center-point (with the net attraction) This would result in those two protons repelling each other for 2/3 of the cycle, because during the remaining time one or the other was acting like a neutron and not participating in mutual electrostatic repulsion. During the electron's 1/6 of the cycle at the center-point, it would cause the electrostatic attraction described above of four times the proton-proton repulsion force. Since this strong electrostatic attraction would then occur for 1/4 as long as the repulsion was present, the net effect would be of exactly canceling out electrostatic (e - p) attraction and (p - p) repulsion forces. This would provide for a stable or meta-stable nucleus.
According to recent NIST data, a Tritium atom has an atomic weight of 3.0160492779 AMU. With a half-life of 12.33 years, it Beta decays into Helium-3, which has an atomic weight of 3.0160293201 AMU.
Those two atomic weights are extremely similar, only 0.0000199578 AMU, which is equivalent to 0.0185906 MeV. This decay produces a Beta- particle (an electron) which has kinetic energy of 0.01859 MeV. So, within an experimental error of around 10 electron-volts, the emitted electron carries away exactly the energy that represents the difference in atomic weights. That seems to imply that there was LESS THAN 0.019 MeV inside the Tritium that could represent the intra-neutron binding energy, a neutrino, and the Strong Force. Within experimental error, this seems to prove that those effects cannot exist within a Tritium atom. Just the binding energy holding a neutron together is far more than that, 0.78235 MeV.
Notice also that the difference in atomic weight between Tritium and Helium-3 is essentially entirely accounted for by the kinetic energy carried away by the escaping electron. Less than 10 electron-volts appears available to account for an escaping neutrino.
There are only two known members of the atomic-weight-3 isotope family, so no parabola can be generated. However the very close exact atomic weights implies a relatively long half-life for Tritium (which is true) and that Helium-3 would be stable (and it is). According to this concept, there are three protons and three electrons in both of these atoms, the only difference being that one of the electrons is in the nucleus in Tritium and orbiting in Helium-3. The extremely small available difference in atomic weight seems to suggest that the total nuclear binding energy for the additional electron inside the nucleus must be 0.0186 MeV. The orbital binding energy of the other, the electron, is 24.6 eV or 0.0000246 MeV, an insignificant factor.
A stable or meta-stable nucleus would certainly require that the net attraction Integral exactly equal the net repulsion Integral, for each nucleon in the nucleus. The brief discussion above hopefully suggests that simple Coulomb forces can explain the observed stabilities of many nuclei.
Even the single 3He exception seems to agree with this premise. There would be three positive protons in the nucleus and one internal electron. That electron would have three necessary locations if the center-point premise is used, while it would provide three times the attractive force during that 1/3 of the time, a similar situation as the 4He nucleus discussed above. Therefore, this premise even provides an explanation for why 3He is the single exception in this category of isotopes.
It therefore appears to explain why Beta- particles (electrons) are generally emitted during the radioactive decay of such excessive-weight isotopes. If one of the excessive number of internal electrons escapes the nucleus, it would appear as the very common Beta- decay. The remaining nucleus would therefore increase in atomic number by one, and would be substantially more stable than before.
In addition to this, the universal extreme symmetry preference for isotopes with even numbers of protons and also even numbers of neutrons seems to suggest a special stability of 4He nuclei, which is the alpha particle. This symmetry-based stability might suggest that such structures exist within heavy nuclei, which might explain why they leave the nucleus as a bundle as an alpha particle. This might imply that within heavy nuclei there are distinct organized structures, the simplest of which would be the alpha particle.
There are many other interesting possible implications of this new premise.
An extremely careful analysis of this graph shows that all nuclei which have even-numbered atomic numbers are very slightly lower than the odd-numbered atomic numbers. For this atomic weight (99), the difference is roughly 0.0004 AMU, on the scale of 400 KeV. This effect is interpreted as a geometrical preference for nuclei that have even-numbers of internal electrons, as implying that a better internal symmetry might exist as compared to if there are an odd number of internal electrons in the nucleus. Such a nucleus has a lower actual atomic weight and therefore a greater resulting binding energy, and is therefore a slightly more stable nucleus.
From this same graph, the slope of the curve at any specific atomic number seems to regularly be reasonably accurate at predicting the negative of the log of the decay half-life; elements farther toward the sides of the graph invariably have extremely short half-lives, all of which result in Beta- decay (left side) or Beta+ decay (right side).
In the case of this graph for atomic weight of 99, only one element is stable, element 44. All of the other isotopes decay radioactively. Element 41 has a half-life of 2.5 minutes; element 42, 67 hours; element 43 has a more complex action, of first 5.9 hours and then 50,000 years; and element 45, 4.5 hours. All of the others have very short half-lives. The low slope of the graph at element 43 suggests that it might be nearly stable, which is somewhat confirmed by the very long half-life of its state after the internal transition. As indicated above, all on the left slope decay by emitting a Beta- and all on the right decay by emitting a positron+.
In the years between 1996 and 2009, I had discovered a number of interesting patterns in four different major fields of Nuclear Physics. Until January 2010, I simply saw them as SEPARATE effects, but which all seemed to hint at some close relationship with each other. I now see that they are actually merely different effects caused by an even larger situation. Under some conditions, the charge of a nucleus can have effects which are dependent on the SQUARE OF THAT CHARGE.
The first compelling effect I had noticed regarding this was regarding the energy levels of a single electron revolving around ionized nuclei of different numbers of charges. I had certainly noticed that the energy held by the single electron in an ionized Helium atom (54.4 eV) was remarkably close to four times that of the single electron in the neutral Hydrogen atom (13.6 eV). I also happened to notice that the ionization energy in the single electron in a doubly-ionized Lithium atom (122.4 eV) is remarkably close to NINE times the Hydrogen ionization. And the single electron orbiting in triply-ionized Beryllium is NINE times. And the single electron orbiting in quad-ionized Boron is SIXTEEN times. And the single electron orbiting in quint-ionized Carbon is TWENTY-FIVE times. And the single electron orbiting in six-ionized Nitrogen is THIRTY-SIX times. and so on all the way through the 32 such ions now known, where the single electron orbiting in 31-times-ionized Germanium is (322 or 1024 times the Hydrogen ionization potential.
The effect is impressively consistent. It also applies to atoms having TWO electrons, and more. At the time (July 2007), I noticed the seeming charge-square relationship but considered that to be too outrageous for credibility! So I assumed that it must be an actual DISTANCE relationship which was somehow caused by the charge differences. A presentation of that data is at Atomic Physics - NIST Atomic Ionization Data Patterns Surprising Patterns in the NIST Data Regarding Atomic Ionization http://mb-soft.com/public3/electroa.html
(This WAS a parabolic shape of the various relationships, which struck me as surprisingly similar to the same-weight-isotope-family parabolas that I had already discovered in 2003)
Soon after that date, my research regarding the so-called Quantum Defect resulted in some interesting results. The Principle Quantum Number of atoms is DEFINED as an INTEGER, but actual experiments have shown that it is not exact. Therefore, a Correction Factor is used in the Rydberg and other formulas, which is called the Quantum Defect. It has traditionally been treated as a Fudge Factor, which was assumed to be un-calculatable. Those formulas involve a SQUARE factor in the denominator, to get even close to correct experimental values. No one seems to have ever analyzed those formulas regarding units. It has apparently been ASSUMED that it MUST BE a distance dimension, but it is not. It is necessarily a CHARGE factor that is squared!
In any case, I had discovered how to present the Quantum Defect as a relatively simple equation. That eliminated it needing to be a Fudge Factor, which, as a Physicist, I found distasteful!
Therefore, this was an entirely different subject that seemed to result in factors that depended on charge-squared. A presentation of that data is at Quantum Defect is NOT a Mathematical Defect- It Can Be Calculated The Quantum Defect is a Physical Quantity and not a Fudge Factor http://mb-soft.com/public3/quantum3.html
Related to THIS research, I discovered that the very bizarre shape of the Mass Defect Chart was actually basically a parabola, where the shape was hidden by the fact that the Mass Defect Chart is generally presented PER ATOMIC WEIGHT rather than as a total. However, the shape was still not a pure parabola, until I realized that it was actually THREE Charts all together. A discussion above shows that when it is divided into the three component Charts, they are then each good parabolas. These Charts also implied a charge-square factor, but less obviously than the above two subjects.
This similarity then hinted that maybe the patterns noted earlier regarding orbiting electrons might also apply to Migrating electrons within the nucleus, specifically the parabolic shape of the energy curve regarding the effect of different numbers of positive charges within the nucleus. These parabolic patterns were immediately noticed, although the parabolas were incomplete and just segments, such as those seen in the three separate Mass Defect charts above. The parabolic pattern implies the charge-squared dependence found in the other effects mentioned here.
In fact, this then suggests that by doing energy analysis, it should be possible to determine EXACT (average) RADII of the locations of Migrating Electrons in the nucleus, for any atom.
These findings therefore showed that the basic assumption I had made around 2003 regarding the parabolic shape of the same-weight-isotope-family charts was not correct, and that the explanation actually was this charge-squared dependence instead. That implied that I needed to examine the NIST data in a slightly different way. Instead of examining same-weight-isotope-families, I needed to examine same-number-of-Migrating-Electrons-families. As noted and shown above, those graphs show even more pure parabolic shapes.
This seems to confirm that the actual basis behind each of these Research findings in recent years is actually a charge-squared dependence of binding energy, whether as orbiting electrons or as internal-nucleus-Migrating-electrons.
Here are some of the complete families of same-number-of-Migrating-Electrons graphs:
Each has a Residual graph that seems to provide some information on aspects of nuclear structure. An example is:
Regarding the apparent charge-squared phenomena that are discussed above, maybe that implies that there is some more compact way to store energy than our current technologies permit. If a large amount of Hydrogen can be ionized and thereby store some amount of electrostatic energy, would similarly ionized Calcium atoms have four hundred times as much energy per number or twenty times as much energy per pound? An interesting thought!
Are there other possible technologies which might somehow benefit from a charge-squared phenomenon? Some day we might know! But such PRACTICAL matters are not really important to Theoretical Nuclear Physicists, where the mere existence of a new phenomena is a wonderful goal.
FOOTNOTE: All Physicists are trained to ONLY submit Research Papers to the Physical Review. I started THIS research in 1996 and I soon realized how extremely important my results were. So I went to all the time and trouble to re-write my Paper in the exact specific FONT and type size that they demanded for acceptance. Separate from my years of Research on these matters, I spent several more months just ensuring that all my i's were dotted and my t's crossed, and all the rest of Formatting that they demanded. I mailed in the (five copies, as I recall) Paper to their address, and waited for a response. There was NONE! I eventually learned that a young man had received my mail, and he was troubled that my work seemed to bring question into the concept of Quantum Dynamics. He made clear that he was a lifelong follower of Quantum, and he DECIDED that my Paper would go no further! What he was SUPPOSED to do was to mail copies of my Paper to five PEERS for them to REVIEW my Paper and Research, such that Peer Review would then determine whether my Paper would get Published. That aberrant young man DENIED my Paper the opportunity to ever be SEEN by any Peer Review! He even told me that he had thrown all the copies of my paperwork into the garbage! He even went further! He told me to NEVER contact the Physical Review ever again!
I felt that the Physical Review Editors could not have been pleased that
a Theoretical Physicist educated at the University of Chicago was denied
even the Peer Review opportunity because of an aberrant young man in their
employ, but I decided to not crowd them on that. Shortly later, I
re-edited my Research into a web-page, which I published in 2003, at:
Nuclear Physics - Statistical Analysis of Isotope Masses Nuclear Structure. (research 1996-2003, published in 2003)
A brief article was added in January 2014, which leaves out much of the
complex stuff and simply presents the basics of the Research and Findings, at:
Nuclear Physics May be Fairly Simple (published Jan 2014)
Conservation of Angular Momentum - An Exception or Violation (Sept 2006)
Galaxy Spiral Arms Stability and Dynamics A purely Newtonian gravitational explanation (Nov 1997, Aug 1998)
Twins Paradox of Relativity Is Absolutely Wrong (research 1997-2004, published Aug 2004)
Perturbation Theory. Gravitational Theory and Resonance (Aug 2001, Dec 2001)
Origin of the Earth. Planetary Gravitational Resonances (Dec 2001)
Rotation of the Sun (Jan 2000)
Origin of the Universe. Cosmogony - Cosmology (more logical than the Big Bang) (devised 1960, internet 1998)
Time Passes Faster Here on Earth than on the Moon (but only a fraction of a second per year!) (Jan 2009)
Globular Clusters. All Globulars Must Regularly Pass Through the cluttered Galaxy Plane, which would be very disruptive to their pristine form. (Nov 1997, Aug 1998)
Existence of Photons. A Hubble Experiment to Confirm the Existence of Individual Photons (experimental proof of quanta) (Feb 2000)
Origin of the Moon - A New Theory (June 2000)
Planetary Rotation of Jupiter, Saturn, and the Earth (Jupiter has a lot of gaseous turbulence which should have slowed down its rapid rotation over billions of years) (March 1998)
Cepheid Variable Stars. Velocity Graph Analysis (Feb 2003)
Compton Effect of Astrophysics. A Possible New Compton Effect (Mar 2003)
Olbers Paradox Regarding Neutrinos (Oct 2004)
Kepler and Newton. Calculations (2006)
Pulsars. Pulsars May Be Quite Different than we have Assumed (June 2008)
Sun and Stars - How the Sun Works - Nuclear Fusion in Creating Light and Heat (Aug 2006)
Stars - How They Work - Nuclear Fusion. Lives of Stars and You (Aug 2004)
Sundial Time Correction - Equation of Time. Sundial to Clock-Time Correction Factor (Jan 2009)
General Relativity - A Moon Experiment to Confirm It. Confirming General Relativity with a simple experiment. (Jan 2009)
General Relativity and Time Dilation. Does Time Dilation Result? (Jan 2009)
Geysers on Io. Source of Driving Energy (June 1998)
Mass Extinction, a New Explanation. A New Explanation for Apparent Periodicity of Mass Extinctions (May 1998, August 2001)
Precession of Gyroscopes and of the Earth. Gyroscope Precession and Precession of the Earth's Equinoxes (Apr 1998)
Ocean Tides - The Physics and Logic. Mathematical Explanation of Tides (Jan 2002)
Earth's Spinning - Perfect Energy Source (1990, Dec. 2009)
Earth's Magnetic Field - Source and Logic. Complex nature of the magnetic field and its source (March 1996)
Earth Spinning Energy - Perfect Energy Source From the Earth's Spinning (1990, Nov. 2002)
Nuclear or Atomic Physics Related Subjects:
Nuclear Physics - Statistical Analysis of Isotope Masses Nuclear Structure. (research 1996-2003, published Nov 2003)
Quantum Defect is NOT a Mathematical Defect- It Can Be Calculated The Quantum Defect is a Physical Quantity and not a Fudge Factor(July 2007)
Atomic Physics - NIST Atomic Ionization Data Patterns Surprising Patterns in the NIST Data Regarding Atomic Ionization (June 2007)
Nuclear Physics - Logical Inconsistencies (August 2007)
Neutrinos - Where Did they all Come From? (August 2004)
Neutrinos - Olbers Paradox Means Neutrinos from Everywhere (Oct 2004)
Quantum Nuclear Physics. A Possible Alternative (Aug 2001, Dec 2001, Jan 2004)
Quantum Physics - Quantum Dynamics. A Potential Improvement (2006)
Quantum Physics is Compatible with the Standard Model (2002, Sept 2006, Oct 2010)
Quantum Dynamics (March 2008)
Ionization Potential - NIST Data Patterns. Surprising patterns among different elements (March 2003)
Mass Defect Chart. (calculation, formula) (research 1996-2003, published Nov 2003)
Assorted other Physics Subjects:
Precession of Gyroscopes and of the Earth. Gyroscope Precession and Precession of the Earth's Equinoxes (Apr 1998)
Earth's Magnetic Field - Source and Logic. Complex nature of the magnetic field and its source (March 1996)
Earth Spinning Energy - Perfect Energy Source (1990, Nov. 2002)
Earth Energy Flow Rates due to Precessional Effects (63,000 MegaWatts) (Sept 2006)
Accurate Mass of the Earth. Gravitational Constant - An Important Gravitation Experiment. (Feb 2004)
Tornadoes - The Physics of How They Operate, including How they Form. Solar Energy, an Immense Source of Energy, Far Greater than all Fossil Fuels (Feb 2000, Feb 2006, May 2009)
Radiometric Age Dating - Carbon-14 Age Determination. Carbon-14, C-14 (Dec 1998)
Mass Extinction, an Old Explanation. An Old Explanation for Apparent Periodicity of Mass Extinctions (Aug 2003)
Hurricanes, the Physics and Analysis A Credible Approach to Hurricane Reduction (Feb 2001)
Sundial Time Correction - Equation of Time. Sundial to Clock-Time Correction Factor (Jan 2009)
http://mb-soft.com/index,html
http://mb-soft.com/public/othersci.html
http://mb-soft.com/index.html